
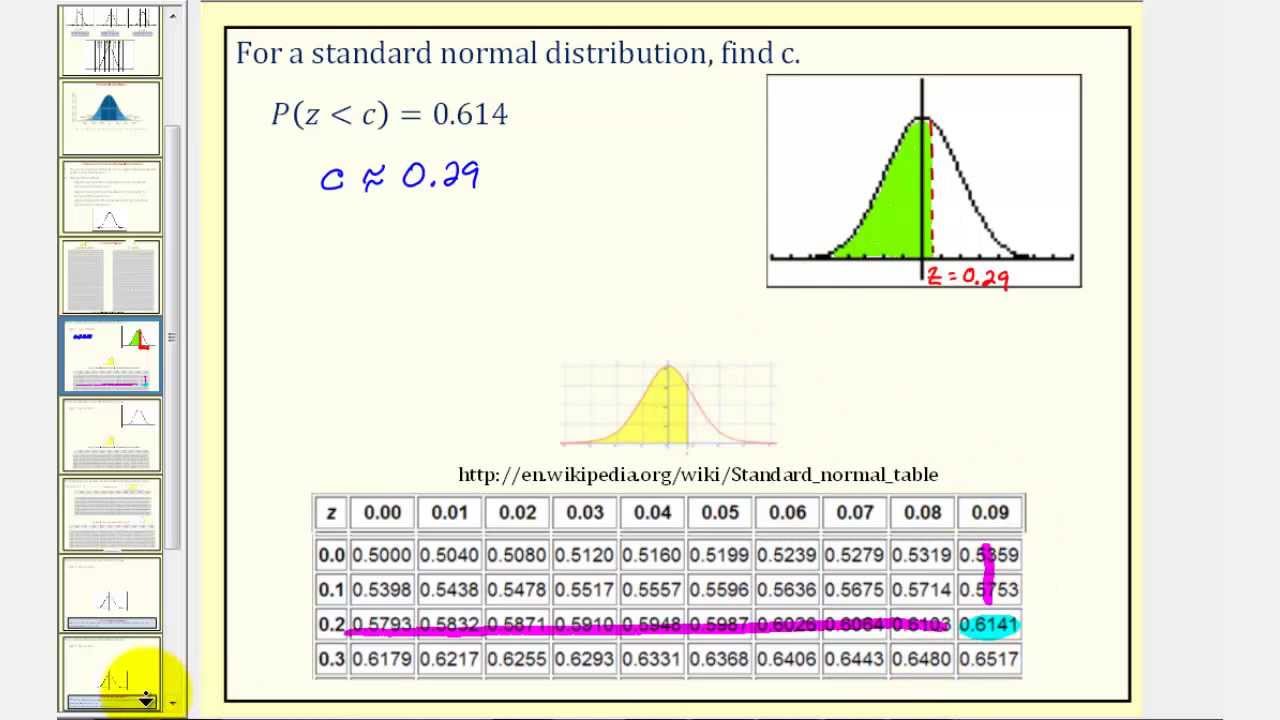
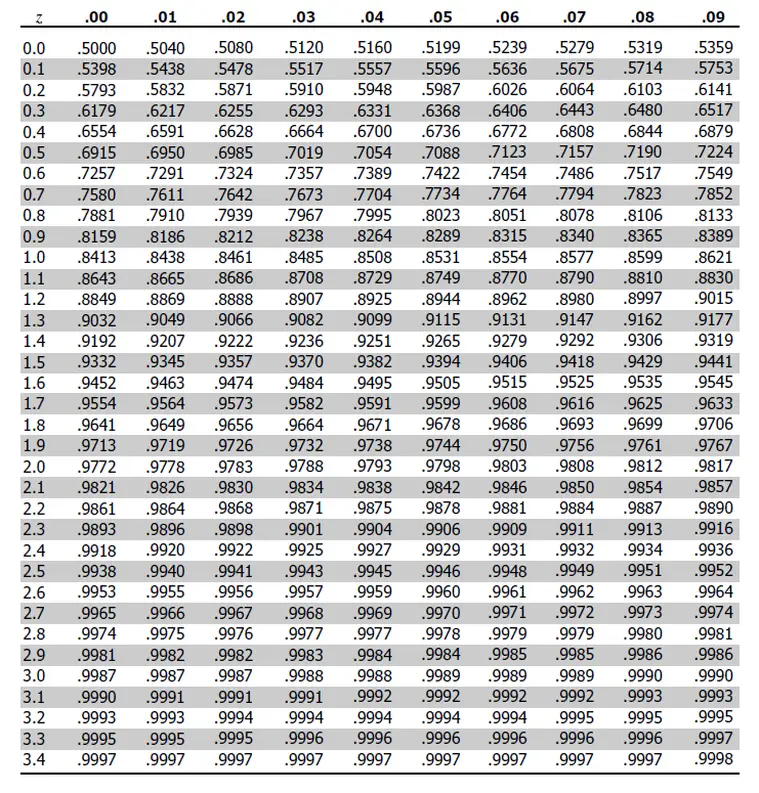
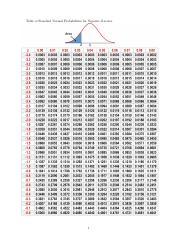
Search the Internet for “standard normal table” and you’ll find hundreds of tables illustrating z-scores and their associated areas. Lucky for us, areas under the N(0, 1) curve can be obtained in numerous other ways, including technology (TI-83/84, Excel) and a table of values. To determine the area under the N (0, 1) curve for any data value that does not fall exactly 1, 2, or 3 standard deviations above or below the mean actually requires some calculus. Finding an Area (Proportion) Given a Specific Z-Value Therefore, even though your actual score on the biology exam was the lowest of the three exam scores, relative to the distribution of all class exam scores, your biology exam score was the highest relative grade. Your statistics exam score was 0.67 standard deviations better than the class average your biology score was 0.7 standard deviations better than the class average your kayaking score was only 0.5 standard deviations better than the class average. Although your highest test score was 91 (kayaking), in which class did you score the best, relative to the rest of the class? We can answer this using a z-score!
Finally, you receive a grade of 91 on your kayaking exam, where the mean grade was 88 and the standard deviation was 6. You receive a grade of 72 on your biology exam, where the mean grade was 65 and the standard deviation was 10.
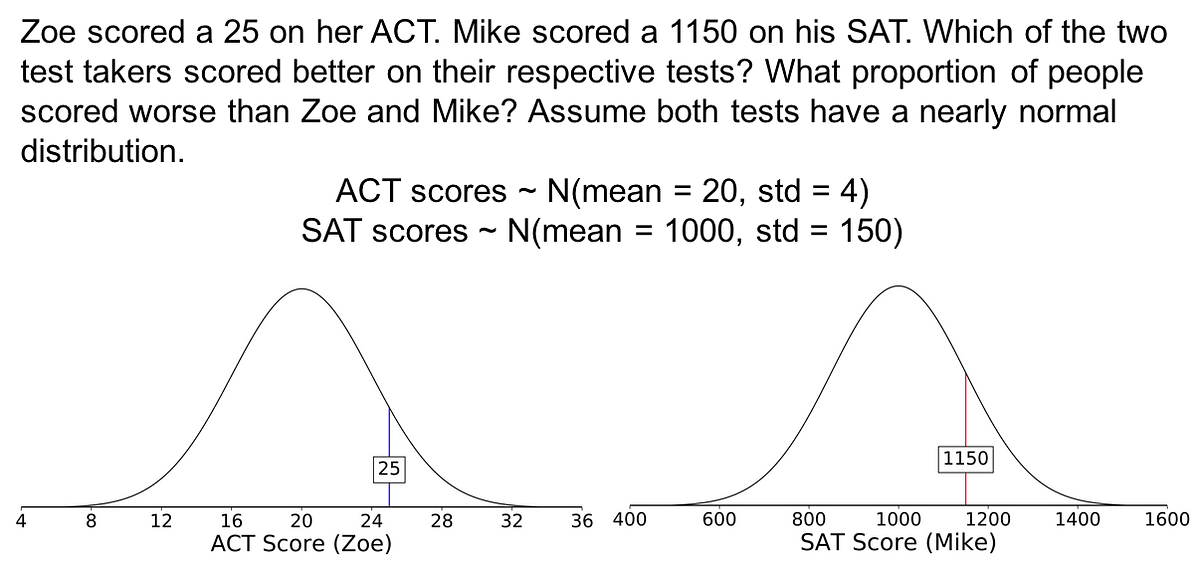
You receive a grade of 82 on your statistics exam, where the mean grade was 74 and the standard deviation was 12. Suppose you are enrolled in three classes, statistics, biology, and kayaking, and you just took the first exam in each. Who, relative to their associated exam, scored better? Example A prime example for this is to compare the test scores for two students, one who scored a 28 on the ACT (scores range from 1 – 36) and another who scored a 1280 on the SAT (scores range from 400 – 1600). Therefore, we’ll be able to compare relative positions of data values within their own distribution to determine which data values are closer to or farther from the mean. In other words, the z-score allows us to standardizetwo or more normal distributions, or more appropriately, to put them on the same scale. Z-scores measure the distance of any data point from the mean in units of standard deviations and are useful because they allow us to compare the relative positions of data values in different samples.
The distribution on the right is a standard normal distribution with a standard score of z = −0.60 indicated. The distribution on the left is a normal distribution with a mean of 48 and a standard deviation of 5. Now draw each of the distributions, marking a standard score of z = −0.60 on the standard normal distribution. Given a normal distribution with μ = 48 and s = 5, convert an x-value of 45 to a z-value and indicate where this z-value would be on the standard normal distribution.īegin by finding the z-score for x = 45 as follows. Obviously a z-score will be positive if the data value lies above (to the right) of the mean, and negative if the data value lies below (to the left) of the mean.Įxample 6.1: Calculating and Graphing z-Values The z-score for any single data value can be found by the formula (in English): But what we’d really like to know is, relative to the spread of our data set, how far is x from μ? Remember that the standard deviation σ gives us a measure of how spread out our entire set of individual data values is. This value will be positive if your data value lies above (to the right) of the mean, and negative if it lies below (to the left) of the mean. If the population standard deviation is available and the sample size is greater than 30, t-distribution can be used with the population standard deviation instead of the sample standard deviation.Given any data value, we can identify how far that data value is away from the mean, simply by doing a subtraction x – μ. Summary: If the sample sizes are larger than 30, the z-distribution and the t-distributions are pretty much the same and either one can be used. The t-score is calculated using the formula: T-Score tells you how many standard deviations from the mean your result is. t-statistics (t-score), also known as Student's T-Distribution, is used when the data follows a normal distribution, population standard deviation ( sigma) is NOT known, but the sample standard deviation ( s) is known or can be calculated, and the sample size is below 30.The z-score is calculated using the formula: Z-Score tells you how many standard deviations from the mean your result is. z-statistics (z-score) is used when the data follows a normal distribution, population standard deviation sigma is known and the sample size is above 30.If you are interested in T-test, you can do similar:


 0 kommentar(er)
0 kommentar(er)
